Fraction Multiplication Techniques and Practice

Slide Note

Utilize visual aids and practice exercises to understand the principles of multiplying fractions, both numerical and algebraic. Explore methods for canceling fractions to simplify calculations efficiently.

jephth Follow

Uploaded on Mar 02, 2025 | 0 Views

Download Presentation

Please find below an Image/Link to download the presentation.

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.

You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.

Download Presentation

The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.

E N D

Presentation Transcript

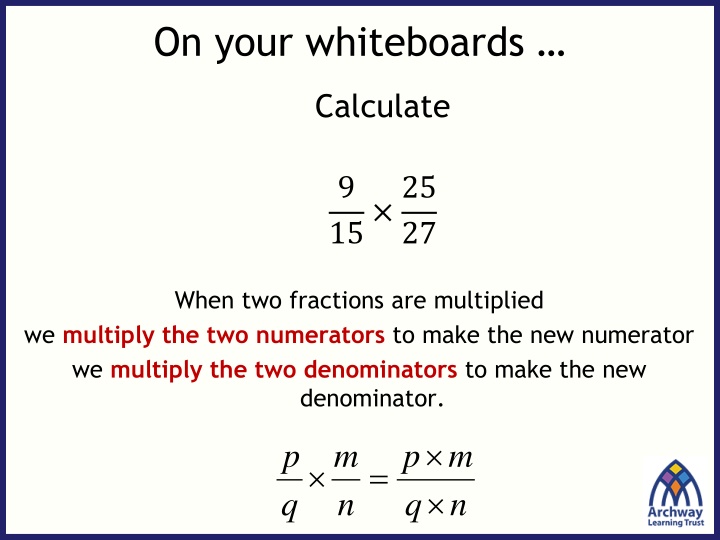
On your whiteboards Calculate 15 25 9 27 When two fractions are multiplied we multiply the two numerators to make the new numerator we multiply the two denominators to make the new denominator. p m p m = q n q n

On your whiteboards 15 25 9 9 25 15 27 = 225 405 27=

Here are 3 methods from another class Are any of them the same as yours? Are any similar? If any are different, can you tell what they have done?

Here are 3 methods from another class Which method is the most efficient?

When we multiply algebraic fractions, we use the same principals as when we multiply numerical fractions. It is usually easier to cancel fractions before multiplying them Remember when cancelling fractions we must cancel a numerator and a denominator

Is there anything we can cancel? 4 5 15 16 Discuss

Is there anything we can cancel? 4? 5 15? 16 What about now?

Is there anything we can cancel? 8 1 3 20 Discuss

Is there anything we can cancel? 8? ?2 3? 20?2 What about now?

Fill in the missing gaps. You may need to cancel first. 7? 8? 16??3 =8??3 5=21?2 7? . . 4) 1) 5 5 10 ?2?2?2 ??? 7? 4 6 . ? =30 . 5) 2) = ???2 5 ?3 7? 7? 21?2 9?=3?2 =3? 27 . . 6) 3) 5 2 ??

Fill in the missing gaps. You may need to cancel first. 3x 7? 8? 16??3 =8??3 5=21?2 7? . . 2 4) 1) 5 5 10 ?2?2?2 ??? 7? 4 6 . ? =30 . 5) 2) = ???2 5 ?3 7? 7? 21?2 9?=3?2 =3? 27 . . 6) 3) 5 2 ??

Fill in the missing gaps. You may need to cancel first. 3x 7? 8? 16??3 =8??3 5=21?2 7? . . 2 4) 1) 5 5 10 5 ?2?2?2 ??? 7? 4 6 . x2 ? =30 . 5) 2) = ???2 5 ?3 7? 7? 21?2 9?=3?2 =3? 27 . . 6) 3) 5 2 ??

Fill in the missing gaps. You may need to cancel first. 3x 7? 8? 16??3 =8??3 5=21?2 7? . . 2 4) 1) 5 5 10 5 ?2?2?2 ??? 7? 4 6 . x2 ? =30 . 5) 2) = ???2 5 ?3 7? 7? 21?2 x2 9?=3?2 =3? 27 . y . 6) 3) 5 2 ??

Fill in the missing gaps. You may need to cancel first. 3x 4 7? 8? 16??3 =8??3 5=21?2 7? . . 2 4) 1) 5 5 5 10 5 ?2?2?2 ??? 7? 4 6 . x2 ? =30 . 5) 2) = ???2 5 ?3 7? 7? 21?2 x2 9?=3?2 =3? 27 . y . 6) 3) 5 2 ??

Fill in the missing gaps. You may need to cancel first. 3x 4 7? 8? 16??3 =8??3 5=21?2 7? . . 2 4) 1) 5 5 5 10 4 5 ?2?2?2 ??? 7? 4 6 . x2 ? =30 . 5) 2) = ???2 5 z ?3 7? 7? 21?2 x2 9?=3?2 =3? 27 . y . 6) 3) 5 2 ??

Fill in the missing gaps. You may need to cancel first. 3x 4 7? 8? 16??3 =8??3 5=21?2 7? . . 2 4) 1) 5 5 5 10 4 5 ?2?2?2 ??? 7? 4 6 . x2 ? =30 . 5) 2) = ???2 5 z ?3 7? 7? 21?2 1 x2 9?=3?2 =3? 27 . y . 6) 3) 5 2 2 ??

Is there anything we can cancel? 3? + 6 ? + 2 ? ?2 Discuss

Is there anything we can cancel? 3(? + 2) ? + 2 ? ?2 What about now? How is this different?

Is there anything we can cancel? 3(? + 2) ? + 2 ? ?2 Sometimes, it helps to factorise some of the expressions to check if we can cancel

Joey and James are trying to simplify the calculation: 5 ? + 2 2 ? Joey thinks that James thinks that ? + 2 2 5 10 ? + 2 2 Because when you multiply 5 10 ? + 2? ?2 + 2? ?= ?= Because x + 2 multiplied by x x + 2 by x you must multiply gives you x + 2x the x and the 2 Discuss who do you agree with?

Joey and James are trying to simplify the calculation: 5 ? + 2 2 ? ? + 2 2 5 10 ?= ?(? + 2) Which method can we use to expand the brackets? ? + 2 2 5 10 ?= ?2+ 2?