Fundamentals of Applying the Method of Fundamental Solutions to Algid Soil Freezing Fronts
Explore the application of the Method of Fundamental Solutions (MFS) in modeling 2-D advancing freezing for algid soil, aiming to enhance technology for estimating heat flux and locating freezing fronts in roadway embankments. The project emphasizes the adaptive and convenient nature of MFS compared to traditional methods, utilizing Matlab for efficient calculations and approximations.
Download Presentation

Please find below an Image/Link to download the presentation.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.If you encounter any issues during the download, it is possible that the publisher has removed the file from their server.
You are allowed to download the files provided on this website for personal or commercial use, subject to the condition that they are used lawfully. All files are the property of their respective owners.
The content on the website is provided AS IS for your information and personal use only. It may not be sold, licensed, or shared on other websites without obtaining consent from the author.
E N D
Presentation Transcript
Applying the Method of Fundamental Solutions to Algid Soil Freezing Fronts ARL-USMA Tech Symposium Samuel Humphries, John Lee, William Nevils, Matthew Fauerbach, Kameron Grubaugh, Bryce Wilkins, Dr. Theodore Hromadka October, 2019
Problem Introduction Goals: Model a 2-D advancing freezing using the method of fundamental solutions (MFS) Upgrade technology Quasi steady-state temperatures along boundary
Problem Motivation Danger posed to roadway embankments Interested in: Locating freezing fronts Estimating heat flux values Advantages of MFS: Adaptive More convenient Advantages of Matlab
Project Process Identify research goals Achieve goals Conference presentation Defend research Continue work
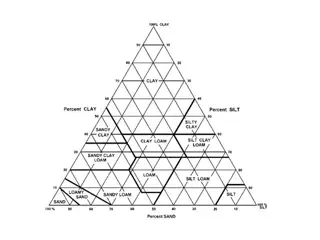
Methodology: Method of Fundamental Solutions Goal: Approximate a function over a given domain from known data points i.e. fluid flow, heat transfer Known points on boundary ??(collocations) Nodes outside problem domain ?? with coefficients ?? Use least squares to solve for ??coefficients to reduce error Approximation function is ?=1 ? ?? ??(??)
Methodology: Application to Freezing Fronts Consider a heat flow problem defined on domain with an exterior boundary Objective equation; solve for s (spatial term): ??? ??= ??? ? Figure: Roadway Embankment Problem and Nodal Placement
Methodology: Application to Freezing Fronts Heat flux across interior given by: ? = ? ? where ?(P) is the flow potential at any point P. For 2-D problems: ? = ??+ ???= ??? ?? ???? ?? ?(P) satisfies the Laplace Equation: 2? ? = 0 Seek to find: ? ? =?? ??= ??(?) and ? ? =?? ??= ??(?) where ? .
Methodology: Application to Freezing Fronts Approximation Function: Choose ?? and ?? to minimize least squares functional ? ???,?;? = ?=1 ??log|?? ?| ? Figure: Roadway Embankment Problem and Nodal Placement
Methodology: Application to Freezing Fronts Velocity Components: (? ??) |?? ?| ? ? = ???,?;? =??? ? ??= ?=1 ?? (? ??) |?? ?| ? ? = ???,?;? =??? ? ??= ?=1 ?? Objective Equation; solve for s: ??? ??= ( ????? ?????? ???= ??) = ?? ? ? ( ????(?) ?????(?)) ?
Anticipated Results Figure: Estimated Freezing Front Locations
Follow-on Work The Conversion Upgrade technology to Matlab Improve computational efficiency Include MFS in conjunction with Complex Variable Boundary Element Method (CVBEM)
Acknowledgments We would like to thank Dr. Hromadka for his continued support and funding on this research topic as well as Bryce Wilkins and Kameron Grubaugh for their help advising our research.